A Scoop of Blancmange
January 02, 2009
The blancmange curve is the set of points $(x, y)$ such that $0 \le x \le 1$ and $y = \sum \limits_{n = 0}^{\infty} {\dfrac{s(2^n x)}{2^n}}$, where $s(x)$ is the distance from $x$ to the nearest integer.
The area under the blancmange curve is equal to ½, shown in pink in the diagram below.
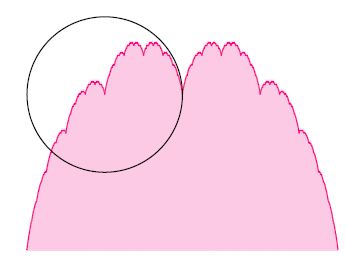
Let C be the circle with centre $\left ( \frac{1}{4}, \frac{1}{2} \right )$ and radius $\frac{1}{4}$, shown in black in the diagram.
What area under the blancmange curve is enclosed by C?Give your answer rounded to eight decimal places in the form 0.abcdefgh
Written by gamwe6 who lives and works in San Francisco building useful things. You should follow him on Twitter